Author: Zebedee Bell
Abstract
This article aims to demystify the Holographic Principle, an emerging theory that encompasses as disparate fields as black hole physics, string theory, cosmology, and quantum mechanics. As such, it is no mean feat to attempt to provide a working knowledge of this theory in a single article. However, it is the author’s goal to illuminate and make accessible this extremely interesting and exciting theory for as many students as possible. This article will examine the origins of the theory and the cascade of discoveries which led to its conception; the current standing of the theory; and its startling implications.
Introduction
The holographic principle is an emerging notion within physics that the universe we know could be viewed as holographic. A hologram is essentially a means of storing the information for a system in a form that exists a dimension lower, like a 3-d image projected from a 2-d film. So, in applying this idea to the entire universe, for however many dimensions there are (string theory being unhelpful in narrowing this down), all the information and mechanics and laws of the universe must be able to be encoded onto a semi-separate system one dimension lower. To make the model visually simpler, it is helpful to imagine the universe as 3-d, in which case there must be a 2-d membrane surrounding this 3-d universe to allow for holography to take place. This 3-d interior is often referred to as the ‘bulk’, and the 2-d external membrane as the ‘boundary’. Now, this theory may sound as if it is edging into sci-fi territory, so how did this seemingly ludicrous theory come to the forefront of science?

Precedent for the Theory

The first notion of holographic space-time emerged from every physicist’s (least) favorite phenomenon: the black hole. Black holes, specifically their nature of being unobservable past the event horizon, keep physicists up at night due to something called the Black Hole Information Paradox [1]. As might be suggested by the name, this paradox is concerned with violating the Conservation of Information, a conservation demanded by quantum mechanics, which means that information cannot be destroyed. Black holes, by the famous no-hair conjecture [2], exhibit only three properties: mass, charge, and angular momentum (pictured right). All the other properties of the particles which fall into the black hole are hidden behind the event horizon. This is not a problem on its own, since the information still exists, it is just not available. However, Hawking [3] demonstrated that black holes evaporate due to Hawking Radiation, which showed that black holes radiate like a black-body emitter, where this radiation is proportional only to the mass (which, and remember this, is proportional to the surface area of a black hole), which means that all the other information locked inside the black hole does not affect Hawking Radiation, meaning that this information is lost when the black hole disappears.

An obvious solution to the paradox is that the information which needs to be conserved escapes via the Hawking Radiation itself. Physicist Jacob Bekenstein [4] realized that if black holes are thermal emitters (hot), then they must have entropy. Entropy is, in essence, the measure of how spread-out energy within a system is. He in fact calculated the entropy of a black hole as:

Where S represents entropy; A is the surface area of the event horizon; c is the speed of light; G is the gravitational constant; and h-bar is the reduced Planck constant.
You may notice that here, as for Hawking Radiation, the entropy of a black hole is proportional to its surface area. Another definition for entropy is that it is the number of micro-states contributing to a macro-state [5], essentially meaning that the event horizon cannot be smooth, but must have a level of disorder. It is possible, therefore, that the event horizon of a black hole is coded like a vinyl record to consist of the entropy, and therefore the information of a black hole. It was Gerard ‘t Hooft [6] who described the mechanism by which in-falling particles can imprint on the event horizon, showing that the in-falling particles cause gravitational deformation, essentially making bumps on the horizon, which totally describe the particle, and so can transfer this information to out-going radiation. This formalized an explanation for how the event horizon can totally describe the contents of the black hole. In other words, the information for the black hole, a 3-d system, is encoded onto the 2-d event horizon (right). From our earlier definition, we can then say that a black hole is a hologram.

Problems were raised with this explanation, however. If information is imprinted in this way on the event horizon, this fits with an outside observer’s perspective since time ceases to exist when approaching the immense gravitational effects of a black hole and so any in-falling objects appear trapped (invisible) on the event horizon. However, from the perspective of the in-falling object, they, and their accompanying information, pass straight through. Assuming the information on the event horizon stays there for all frames of reference, this then raises a problem since it violates the no-cloning theorem, whereby information cannot be duplicated in this way. Leonard Susskind [7] replied to this criticism by inventing the idea of black hole complementarity. You have likely heard of the Heisenberg Uncertainty Principle in the context that we cannot know both the momentum and position of a system with 100% certainty at the same time. We say that momentum and position have complementarity. There is another form of complementarity in energy and time, in which the conservation of energy can actually be broken if it is only for a very short time. For example, an electron-positron pair can appear out of a vacuum and then almost immediately annihilate (within about 10^-40 seconds), without breaking the laws of nature. Similarly, in black hole complementarity, the no-cloning theorem can be “broken” by saying that since it is impossible to observe both the exterior and interior of a black hole at the same time, information can be duplicated on the event horizon [8].
Extrapolation
Okay, sure, but black holes are really weird things, so how can physicists possibly say from this that we can extend the notion of black hole holography to the entire universe? Foremostly, in calculating his equation for black hole entropy, Bekenstein [9] worked out the maximum information that can be stored within the black hole, finding it proportional to the black hole surface area. Well, Bekenstein also extrapolated this principle into Bekenstein Bounds which measured the maximum information storage of any space, finding it again proportional to the surface area of the enclosed space. Moreover, similar (in that it is exactly opposite) to the black hole’s event horizon is a boundary in our observable universe beyond which the universe is accelerating faster than the speed of light (the universe’s expansion accelerating away from each point in space), making it unobservable relative to our position, very akin to the event horizon, only pushing outwards rather than pulling inwards [10].
A black hole to the universe is still, however, a gigantic leap, so now, for the missing connections. It is easy to imagine a black hole since it is necessarily a spherical object with finite bounds. The universe, however, is a little bit harder to imagine. There are essentially three contenders, which revolve around what the value is for the curvature of space-time. If the value is positive, then space-time curves in on itself, forming a finite sphere in which two parallel lines will eventually converge; this is known as de-Sitter space [11]. If this value for curvature is zero, then space-time is a perfectly flat plane in which two parallel lines will stay parallel. The final, and hardest, to imagine is where the curvature is negative, in which case the universe is a sort of saddle shape, or a hyperbolic space-time, where initially parallel lines will diverge; this is known as anti- de Sitter space (AdS) [12]. Now, it is difficult to imagine any sort of coordinate system for mapping AdS space-time; various ‘compactifications’ have been formulated which, like how the spherical world can be compressed onto a 2-d plane, try to map the infinite hyperbolic universe onto a 2-d plane. One of the best models was created (not for researching the holography principle) by mathematician Poincare [13]. As shown left, the Poincare board conformally (meaning the angles remain the same) compactifies hyperbolic space-time onto a circle in which the tessellation pattern becomes increasingly smaller towards the infinite boundaries. Though difficult to visualise, if the centre of the board were to be shifted to the right, with the right side therefore enlarging and the left side growing, the pattern remains exactly the same; in other words, the shape has the same observed boundaries regardless of the point of reference. This fits with our notion of the cosmic light horizon. Even more exciting is the fractal nature of the Poincare board since any hologram must have a fractal nature in that the entire image is contained within any section of the hologram.

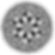
The connection was made at last by Juan Maldecena [14] who formulated the idea of Ads/CFT correspondence. CFT stands for conformal field theory, where the rules which govern interactions within a field are scale invariant, so remain the same at whatever scale. Another common application of scale invariance is in string theory, where the vibrating strings theorized to constitute all matter behave the same at whatever length/energy they are at. Maldecena imagined a series of stacked, almost overlapping, d-branes (objects in string theory of variable dimensionality which close open strings), connected by scale-invariant strings, and he found that this produced a conformal field theory in line with quantum mechanics, behaving like Minkowski space-time [15]. The next step was for him [16] to imagine these brane structures in AdS space, where a cylinder is formed from the Poincare board being given height by time (right). He found that the surface of this cylinder behaved exactly like Minkowski space-time as before, with the CFT for quantum theory still present, while the interior also demonstrated a field theory which incorporated gravity. He had, in essence, formulated a holographic view of space-time in which interactions from the CFT on the lower dimensional ‘boundary’ can project a set of laws onto the AdS ‘bulk’. As a note, it is helpful conceptually to restrict models to three spatial dimensions, but Maldacena’s calculations necessitate that the boundary be four-dimensional, and the bulk be five-dimensional [17].

Implications
Perhaps the most startling prospect of the holographic principle is finally formulating a theory for quantum gravity. After all, the AdS/CFT correspondence does demonstrate a system in which quantum mechanics and gravity can be described in a unified model [18]. Considering the universe as a hologram allows physicists to consider gravity as a projection of quantum mechanics in a higher dimension. This is especially useful due to the inverse correspondence between the boundary and the bulk, in that extremely large gravitational phenomena in the bulk (like black holes) become far easier to calculate from when looked at in the boundary, where the event becomes very weak. Similarly, extremely complex quantum systems become far easier to deal with when viewed as their projected form in the bulk, becoming far simpler. Another intriguing possibility which arises from the holographic nature of the universe comes from the fractal properties of holograms mentioned earlier. The bulk, as would be expected, is not a fractal since it has an integer dimensionality of 2 (calculated from a number of red-shift surveys) [19], but the holographic ‘film’ itself, the boundary may be. If the boundary, and therefore the universe’s quantum mechanical interactions are fractal in nature, this provides an intriguing explanation for quantum entanglement that particles become entangled because they are simply the self-similar repeating patterns of one another. This could be extrapolated further to support the one-electron theory [20] which states that each electron in the universe has the same mass and charge because it is actually just the same electron. The traditional explanation is that electrons could be deflected backwards and forwards through time to result in this, but perhaps a more elegant theory is that each electron is essentially the same because they are all segments of a fractal nature to the universe on a quantum scale.
Conclusion
The holographic principle is a theory which arose from decades of mathematical interpretation and theoretical exploration, a collaboration between some of the strangest and most apparently disparate fields. It is a theory with cosmic-scale implications to the way we understand physics and the way in which we consider our own place within the universe. The theory is not without its problems and its contenders, but it is the culmination of some of the greatest minds of our generation which has led to what may be one of the most drastic cosmological revolutions in our history. It is my hope that this article has served to elucidate this intriguing field and to have provided a sufficient level of knowledge to allow for a profound understanding of this theory.
Bibliography
1. Polchinski, J. “The Black Hole Information Problem”. New Frontiers in Fields and Strings (TASI 2015)
2. “Leonard Susskind on The World As Hologram”. YouTube video, 55:26. Posted by “TVO Docs”, 4 Nov 2011
3. Hawking, S.W. “Particle creation by black holes”. Commun.Math. Phys. 43, 199–220 (1975).
4. Bekenstein, J. “Black Holes and Entropy”. American Physical Society, 1973
5. Thibault, D. “The Entropy of Black Holes: A Primer” Progress in Mathematical Physics, Volume 38. (2004)
6. ‘t Hooft, G “The Good, the Bad, and the Ugly of Gravity and Information”. arXiv: High Energy Physics – Theory (2016):
7. “Leonard Susskind on The World As Hologram”
8. Carlip, S. “Black Hole Thermodynamics”. International Journal of Modern Physics D, Volume 23, Issue 11 (2014)
9. Bekenstein “Black Holes and Entropy”
10. “Leonard Susskind on The World As Hologram
11. “Understanding the Holographic Universe”. YouTube playlist, 2:02:26. Posted by PBS Space Time, Last Updated 25 Oct 2019
12. Witten, E “Anti de Sitter Space and Holography”. Advances in Theoretical and Mathematical Physics vol. 2 (1998): 253-291
13. “Understanding the Holographic Universe
14. Maldacena, J. “The Large-N Limit of Superconformal Field Theories and Supergravity”. International Journal of Theoretical Physics 38, 1113–1133 (1999). https://doi.org/10.1023/A:1026654312961
15. Witten, “Anti de Sitter Space and Holography”
16. Maldacena “The Large-N Limit of Superconformal Field Theories and Supergravity”
17. Maldacena “The Large-N Limit of Superconformal Field Theories and Supergravity”
18. Suvrat. R. “Lessons from the Information Paradox” eprint arXiv:2012.05770 (2020)
19. Mureika J. “Fractal holography: a geometric re-interpretation of cosmological large-scale structure”. Journal of Cosmology and Astroparticle Physics Vol. 2007
20. “Understanding the Holographic Universe”
Figure References
Figure 1 – Popular Perception of Holography, credit: Amy Richau “6 Ways Holograms Play an Important Role in Star Wars Storytelling”
Figure 2 – Visualisation of No-Hair Conjecture, credit: Norman Gürlebeck “No-Hair Theorem for Black Holes in Astrophysical Environments”
Figure 3 – Black Body Emission Spectrum, credit: Encyclopaedia Britannica, Inc.
Figure 4 – Bekenstein’s Black Hole Entropy Equation, credit: Brian Greene, @bgreene on Twitter 15 March 2018
Figure 5 – Visualisation of ‘t Hooft’s Entropy Solution, credit: vystavil luboš motl v “Hawking, Perry, Strombinger on soft hair”
Figure 6 – Euclidian and Non-Euclidian Geometry, credit: naidseyes “The use of non-Euclidian geometry in art”
Figure 7 – Poincare Board, credit: Weisstein, Eric W. \”Poincaré Hyperbolic Disk.\” From MathWorld–A Wolfram Web Resource. https://mathworld.wolfram.com/PoincareHyperbolicDisk.html
Figure 8 – AdS/CFT correspondence, credit: Alex Dunkel (based off an image in Maldacena, J “The Illusion of Gravity” (2005))
